| Super-types: | AbstractCurveSegmentType < CubicSplineType (by extension) |
|---|---|
| Sub-types: | None |
| Name | CubicSplineType |
|---|---|
| Abstract | no |
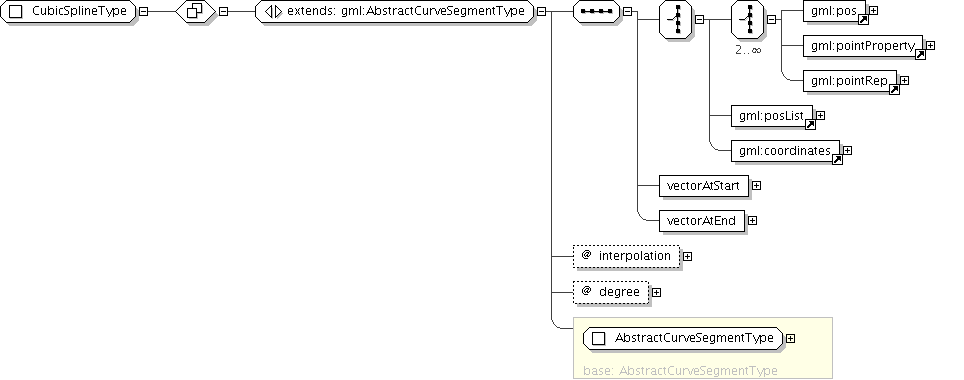
| Documentation | Cubic splines are similar to line strings in that they are a sequence of segments each with its own defining function. A cubic spline uses the control points and a set of derivative parameters to define a piecewise 3rd degree polynomial interpolation. Unlike line-strings, the parameterization by arc length is not necessarily still a polynomial. The function describing the curve must be C2, that is, have a continuous 1st and 2nd derivative at all points, and pass through the controlPoints in the order given. Between the control points, the curve segment is defined by a cubic polynomial. At each control point, the polynomial changes in such a manner that the 1st and 2nd derivative vectors are the same from either side. The control parameters record must contain vectorAtStart, and vectorAtEnd which are the unit tangent vectors at controlPoint[1] and controlPoint[n] where n = controlPoint.count. Note: only the direction of the vectors is relevant, not their length. |
'The attribute \"numDerivativesAtStart\" specifies the type of continuity between this curve segment and its predecessor. If this is the first curve segment in the curve, one of these values, as appropriate, is ignored. The default value of \"0\" means simple continuity, which is a mandatory minimum level of continuity. This level is referred to as \"C 0 \" in mathematical texts. A value of 1 means that the function and its first derivative are continuous at the appropriate end point: \"C 1 \" continuity. A value of \"n\" for any integer means the function and its first n derivatives are continuous: \"C n \" continuity. NOTE: Use of these values is only appropriate when the basic curve definition is an underdetermined system. For example, line string segments cannot support continuity above C 0 , since there is no spare control parameter to adjust the incoming angle at the end points of the segment. Spline functions on the other hand often have extra degrees of freedom on end segments that allow them to adjust the values of the derivatives to support C 1 or higher continuity.'
"'The attribute \"numDerivativesAtEnd\" specifies the type of continuity between this curve segment and its successor. If this is the last curve segment in the curve, one of these values, as appropriate, is ignored. The default value of \"0\" means simple continuity, which is a mandatory minimum level of continuity. This level is referred to as \"C 0 \" in mathematical texts. A value of 1 means that the function and its first derivative are continuous at the appropriate end point: \"C 1 \" continuity. A value of \"n\" for any integer means the function and its first n derivatives are continuous: \"C n \" continuity. NOTE: Use of these values is only appropriate when the basic curve definition is an underdetermined system. For example, line string segments cannot support continuity above C 0 , since there is no spare control parameter to adjust the incoming angle at the end points of the segment. Spline functions on the other hand often have extra degrees of freedom on end segments that allow them to adjust the values of the derivatives to support C 1 or higher continuity.'
"'The attribute \"numDerivativesInterior\" specifies the type of continuity that is guaranteed interior to the curve. The default value of \"0\" means simple continuity, which is a mandatory minimum level of continuity. This level is referred to as \"C 0 \" in mathematical texts. A value of 1 means that the function and its first derivative are continuous at the appropriate end point: \"C 1 \" continuity. A value of \"n\" for any integer means the function and its first n derivatives are continuous: \"C n \" continuity. NOTE: Use of these values is only appropriate when the basic curve definition is an underdetermined system. For example, line string segments cannot support continuity above C 0 , since there is no spare control parameter to adjust the incoming angle at the end points of the segment. Spline functions on the other hand often have extra degrees of freedom on end segments that allow them to adjust the values of the derivatives to support C 1 or higher continuity.'
"'The attribute \"interpolation\" specifies the curve interpolation mechanism used for this segment. This mechanism uses the control points and control parameters to determine the position of this curve segment. For a CubicSpline the interpolation is fixed as \"cubicSpline\".'
"'The degree for a cubic spline is \"3\".'
">'GML supports two different ways to specify the control points of a curve segment. 1. A sequence of \"pos\" (DirectPositionType) or \"pointProperty\" (PointPropertyType) elements. \"pos\" elements are control points that are only part of this curve segment, \"pointProperty\" elements contain a point that may be referenced from other geometry elements or reference another point defined outside of this curve segment (reuse of existing points). 2. The \"posList\" element allows for a compact way to specifiy the coordinates of the control points, if all control points are in the same coordinate reference systems and belong to this curve segment only. The number of direct positions in the list must be at least three.'
'Deprecated with GML version 3.1.0. Use \"pointProperty\" instead. Included for backwards compatibility with GML 3.0.0.'
'Deprecated with GML version 3.1.0. Use \"posList\" instead.'
'"vectorAtStart" is the unit tangent vector at the start point of the spline.'
'"vectorAtEnd" is the unit tangent vector at the end point of the spline.'
